Mauchly’s test of sphericity is used to test whether or not the assumption of sphericity is met in a repeated measures ANOVA.
Sphericity refers to the condition where the variances of the differences between all combinations of related groups are equal.
If this assumption is violated, then the F-ratio becomes inflated and the results of the repeated measures ANOVA become unreliable.
How to Perform Mauchly’s Test of Sphericity
Mauchly’s test of sphericity uses the following null and alternative hypotheses:
- H0: The variances of the differences are equal
- HA: The variances of the differences are not equal
If the p-value of the test is less than some significance level (e.g. α = .05) then we reject the null hypothesis and conclude that the variances of the differences are not equal.
Otherwise, if the p-value is not less than some significance level (e.g. α = .05) then we fail to reject the null hypothesis and conclude that the assumption of sphericity is met.
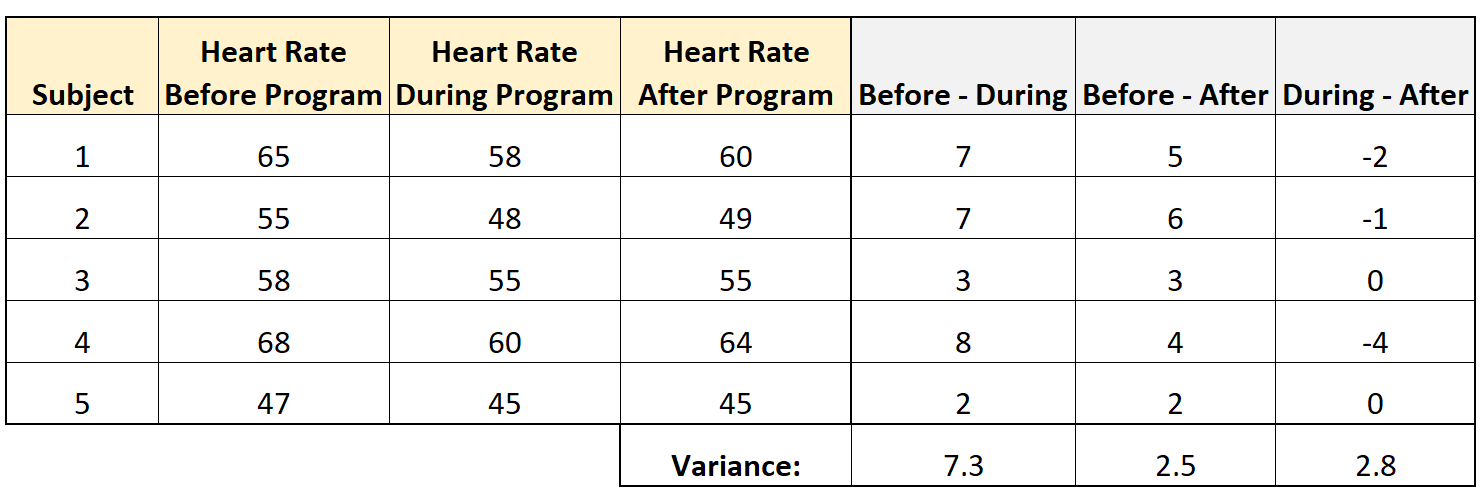
For example, suppose a doctor measures the resting heart rate of subjects during three different time points:
- One month before starting a training program
- In the middle of a training program
- One month after a training program
He wants to perform a repeated measures ANOVA to see if there is a significant difference in mean resting heart rate across these three time points.
The following table shows the results of his data collection:
We can see that the variances of the differences are not all equal.
To determine if these differences are statistically significant, we can perform Mauchly’s test of sphericity using some statistical software like R, SPSS, Python, etc.
Depending on which software you use, the results of the test will look something like this:
We would then report the results of the test as follows:
Mauchly’s test of sphericity indicates that the assumption of sphericity has not been violated, X2(2) = 1.867, p = .356.
What to Do if Sphericity is Violated
In scenarios where the p-value is less than .05 and we reject the null hypothesis of Mauchly’s test of sphericity, we typically apply a correction to the degrees of freedom used to calculate the F-ratio.
There are three corrections we can apply:
- Huynh-Feldt (least conservative)
- Greenhouse–Geisser
- Lower-bound (most conservative)
Each of these corrections tend to increase the p-values in the output table of the repeated measures ANOVA to account for the fact that the assumption of sphericity is violated.
Additional Resources
The following tutorials provide additional information on how to perform a Repeated Measures ANOVA:
An Introduction to the Repeated Measures ANOVA
How to Report the Results of a Repeated Measures ANOVA
One-Way Repeated Measures ANOVA Calculator