This tutorial explains the differences between the statistical methods ANOVA, ANCOVA, MANOVA, and MANCOVA.
ANOVA
An ANOVA (“Analysis of Variance”) is used to determine whether or not there is a statistically significant difference between the means of three or more independent groups. The two most common types of ANOVAs are the one-way ANOVA and two-way ANOVA.
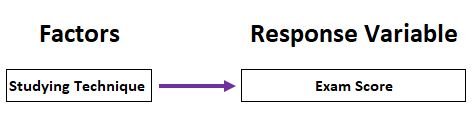
One-Way ANOVA: Used to determine how one factor impacts a response variable.
Example: You randomly split up a class of 90 students into three groups of 30. Each group uses a different studying technique for one month to prepare for an exam. At the end of the month, all of the students take the same exam. You want to know whether or not the studying technique has an impact on exam scores so you conduct a one-way ANOVA to determine if there is a statistically significant difference between the mean scores of the three groups.
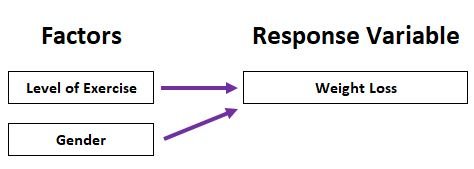
Two-Way ANOVA: Used to determine how two factors impact a response variable, and to determine whether or not there is an interaction between the two factors on the response variable.
Example: You want to determine if level of exercise (no exercise, light exercise, intense exercise) and gender (male, female) impact weight loss. In this case, the two factors you’re studying are exercise and gender and your response variable is weight loss (measured in pounds). You can conduct a two-way ANOVA to determine if exercise and gender impact weight loss and to determine if there is an interaction between exercise and gender on weight loss.
ANCOVA
An ANCOVA (“Analysis of Covariance”) is also used to determine whether or not there is a statistically significant difference between the means of three or more independent groups. Unlike an ANOVA, however, an ANCOVA includes one or more covariates, which can help us better understand how a factor impacts a response variable after accounting for some covariate(s).
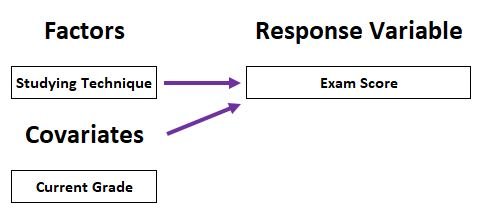
Example: Consider the same example we used in the One-Way ANOVA. We split up a class of 90 students into three groups of 30. Each group uses a different studying technique for one month to prepare for an exam. At the end of the month, all of the students take the same exam.
We want to know whether or not the studying technique has an impact on exam scores, but we want to account for the grade that the student already has in the class so we use their current grade as a covariate and conduct an ANCOVA to determine if there is a statistically significant difference between the mean scores of the three groups.
This allows us to test whether or not studying technique has an impact on exam scores after the influence of the covariate has been removed. Thus, if we find that there is a statistically significant difference in exam scores between the three studying techniques, we can be sure that this difference exists even after accounting for the students current grade in the class (i.e. if they’re already doing well or not in the class).
MANOVA
A MANOVA (“Multivariate Analysis of Variance”) is identical to an ANOVA, except it uses two or more response variables. Similar to the ANOVA, it can also be one-way or two-way.
Note: An ANOVA can also be three-way, four-way, etc. but they are less common.
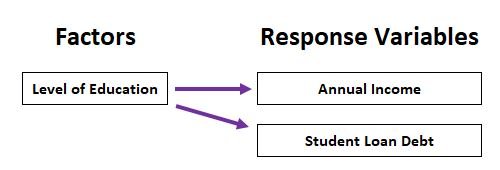
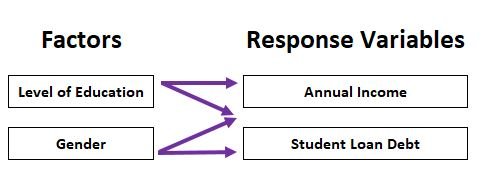
One-Way MANOVA Example: We want to know how level of education (i.e. high school, associates degree, bachelors degrees, masters degree, etc.) impacts both annual income and amount of student loan debt. In this case, we have one factor (level of education) and two response variables (annual income and student loan debt), so we need to conduct a one-way MANOVA.
Two-Way MANOVA Example: We want to know how level of education and gender impacts both annual income and amount of student loan debt. In this case, we have two factors (level of education and gender) and two response variables (annual income and student loan debt), so we need to conduct a two-way MANOVA.
MANCOVA
A MANCOVA (“Multivariate Analysis of Covariance”) is identical to a MANOVA, except it also includes one or more covariates. Similar to a MANOVA, a MANCOVA can also be one-way or two-way.
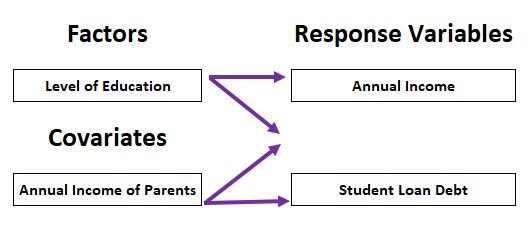
One-Way MANCOVA Example: We want to know how a students level of education impacts both their annual income and amount of student loan debt. However, we want to account for the annual income of the students parents as well. In this case, we have one factor (level of education), one covariate (annual income of the students parents) and two response variables (annual income of student and student loan debt), so we need to conduct a one-way MANCOVA.
Two-Way MANCOVA Example: We want to know how a students level of education and their gender impacts both their annual income and amount of student loan debt. However, we want to account for the annual income of the students parents as well. In this case, we have two factors (level of education and gender), one covariate (annual income of the students parents) and two response variables (annual income of student and student loan debt), so we need to conduct a two-way MANCOVA.